Sunspots on a rotating Sun Teach article
Explore simple harmonic motion with real astronomical images.
In this journal a few years ago, I described using Jupiter’s moons to explore simple harmonic motion and mentioned that I would like to extend the project internationally (Ribeiro, 2012). This came to pass, but using a different astronomical phenomenon – sunspots. In 2013 and 2014, students from France, Greece, Italy, Poland, Portugal, Romania and Spain participated in an eTwinning projectw1 to determine the Sun’s rotational period using sunspots. They designed their own experiments, prepared reports and worked with real data from the Solar and Heliospheric Observatory (SOHO) database maintained by NASAw2.
Based on their work, this activity explains how to use equations relating to simple harmonic motion and sunspot images with a class of students aged 16-19. The main activity lasts approximately one hour but there are also extension ideas for a multi-lesson project, and you could even set up a network of classes similar to ours.
Sunspots and simple harmonic motion
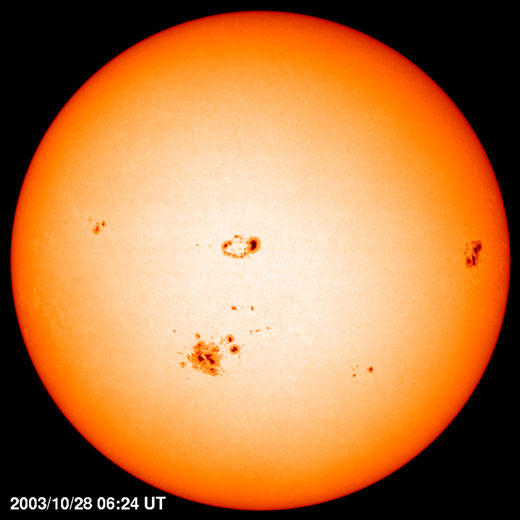
Sunspots are temporary dark spots on the surface of the Sun. If we assume that they have no motion, we can use them to track the Sun’s rotation.
As seen from Earth, a sunspot shows simple harmonic motion. Simple harmonic motion (SHM) is the term used to describe regular periodic motions such as the swinging of a pendulum or the oscillations of an object attached to a spring.
For this experiment you can use images of sunspots taken by SOHO (Solar Heliospheric Observatory), a space-based observatory that investigates the Sun from its deep core, through its atmosphere and the domain of the solar wind.
Materials
- Several images of the same sunspot taken at different times, obtained from SOHO. During our project we used images taken one day apart.
- Ruler
- Pencil
- Graph paper
- Calculator
Procedure
- If you are running this experiment as part of a larger project, you could begin as we did by asking your students to research the Sun and sunspots: what they are, and perhaps their link to Earth’s climate and aurorae.
The Sun, like all stars, is a globe of hot plasma – mainly ionised hydrogen undergoing fusion. This fusion releases energy in the middle of the Sun that is released as electromagnetic radiation by the atmosphere. As a result, the Sun glows.
Sunspots occur when concentrations of the Sun’s magnetic field inhibit the convection of the charged plasma. This lower convection reduces the surface temperature. Temperatures in the dark centres of sunspots drop to about 3700 K (compared with 5700 K for the rest of the Sun’s surface) and so the surface appears much darker.
- To determine the Sun’s rotational period using images of a sunspot from the SOHO database, each group should measure the displacement (x) – the distance of their sunspot from the central axis of the Sun – at a succession of times (t) with a ruler.
- Groups should record their data in a table and note the maximum possible displacement (the distance from the rotational axis to the outside edge of the Sun’s image at the latitude of the sunspot).
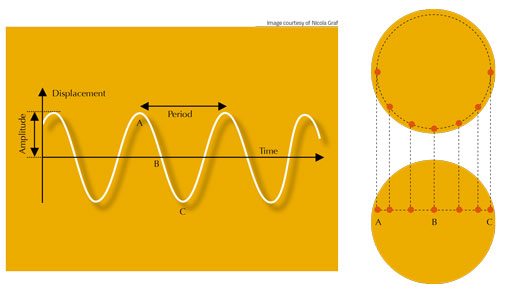
SHM can be interpreted as a projection onto one axis of an object that is moving with a uniform circular motion (UCM). In other words an object moving in a circle in the horizontal plane viewed from the side (equivalent to projection onto the x axis) will show the same to-and-fro motion as an oscillating object attached to a spring. Only when viewing the motion from above would we see the movement as circular. Plotting a graph of this motion (distance from the central point against time) produces the characteristic form of a sine wave (figure 1).
Right hand image courtesy of Carla Isabel Ribeiro
This is what should be apparent when measuring the horizontal displacement of the sunspots; they appear to move faster near the rotational axis at the centre of the Sun and slower as they approach the outer edges, as if they were attached to a spring. The relationship between the two motions (SHM and UCM) is represented by the equation:
x = Asin(ω t + φ) (1)
Where x and A are the displacement and the maximum displacement on the linear trajectory of the sunspot as seen from Earth, respectively. The physical quantities ω and φ are the angular frequency and the phase constant of the circular motion when seen from the solar pole, respectively.
Because angular frequency ω = 2π / T, where T is the orbital period, equation 1 can be rewritten as:
x = Asin[(2π / T) t + φ] (2)
arcsin(x / A) = (2π / T)t + φ (3)
- The students should calculate arcsin(x/A) for all of their measured values of x and then plot a graph of arcsin(x/A) against t (figure 2).
- They should then draw a line of best fit for the data.
- Measuring the gradient of the line will give the rotational period of the Sun. In the example above (figure 2), the gradient is 0.01 radians per hour and so T = 2π / ω = 628.3 hours = 26 days.
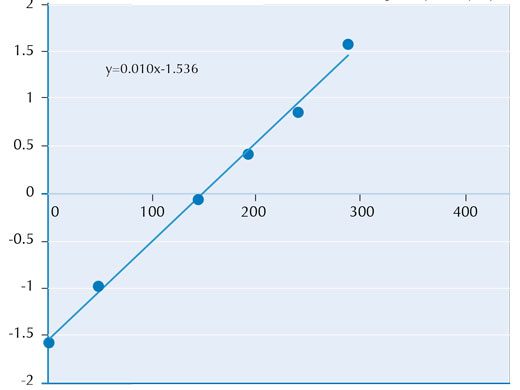
Image courtesy of Nikos Kyriazopulos
Extending the activity
If you wish to extend this activity, each student can repeat the experiment using images of a sunspot of their choice. This time, they should also measure the sunspot’s latitude, allowing them to compare the Sun’s rotation at different latitudes by comparing their results with those of other students.
Measuring the rotation at different latitudes should show that the Sun has a differential rotation: because it is not solid, the rotational period near the poles (about 34 days) is more than at the equator (about 25 days). Discuss why this might be with your students
Can you think of how to improve this project or would you like to set up a similar European network with other schools? We encourage you to comment on the online version of this article.
References
- Ribeiro C (2012) Galileo and the moons of Jupiter: exploring the night sky of 1610. Science in School 25: 41–47.
Web References
- w1 – The eTwinning portal is run and managed by European Schoolnet and is a virtual meeting point for the exchange of information between schools.
- w2 – You can download real-time data from the Solar and Heliospheric Observatory (SOHO). SOHO is a project of international cooperation between the European Space Agency and the US National Aeronautics and Space Administration.
- w3 – The different methods used by the students during the project are described by the students themselves on the project’s MagazineFactory site.
Resources
- In our project the participants used different methods to measure the displacement of the sunspots, from simply using a ruler or drawing a grid onto the images of the Sun to using image processing software, and these are described on the project websitew3.
- Wikimedia Commons hosts several sunspot videos including two tracking the formation and growth of sunspots on the rotating Sun. See http://commons.wikimedia.org or use the direct links.
- The Alienworlds website from the University of South Wales has an animation that explains the Sun and sunspots. See http://alienworlds.southwales.ac.uk or use the direct link.
Review
As with the stars of the night sky, too few young people today have seen sunspots – although they are fascinating and have played a role in the development of the science of astronomy. (If you’d like to see them for yourself rather than using the pictures here, view the Sun through well darkened glass or by projection through a pinhole. Take care: looking directly at the Sun can cause eye damage. Note, though, that sometimes there are few spots for months or even years.)
If you measure the horizontal position of a given sunspot as the days pass, you will have a fraction of a cycle of simple harmonic motion. In the article, this information is used to work out the Sun’s period of rotation with a surprising result.
Eric Deeson, UK





